Ratio Test
- Видео
- О видео
- Скачать
- Поделиться
Ratio Test
791, 656 | 7 год. назад | 8, 297 - 0
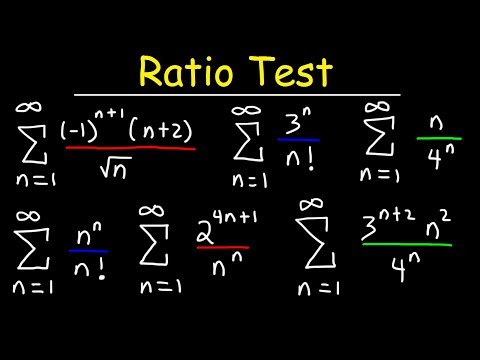
This calculus 2 video tutorial provides a basic introduction into the ratio test. Examples include the ratio test with factorials, exponents, fractions, and square roots. If the limit of the ratio of next term with the previous is less than 1, the series converges. If it's more than 1, the series divergence. If the limit equals 1, the ratio test is inconclusive.
Integral Test For Divergence:
Remainder Estimate - Integral Test:
P-Series:
Direct Comparison Test:
Limit Comparison Test:
_________________________________
Alternating Series Test:
Alternate Series Estimation Theorem:
Absolute & Conditional Convergence:
The Ratio Test:
The Root Test:
__________________________________
Series Tests - Practice Problems:
Taylor & Maclaurin Polynomials:
Taylor's Remainder Theorem:
Power Series - Interval Convergence:
Power Series - Derivatives & Integrals:
___________________________________
Calculus Final Exam and Video Playlists:
Full-Length Videos and Worksheets:
Чтобы скачать видео "Ratio Test" передвинте ползунок вправо
- Комментарии
Комментарии ФБ